Good Financial Planning can be worth its weight in gold but how do you define the actual value of advice in the investment process, the so-called "Gamma" factor that adds extra performance to a portfolio through picking the right funds and avoiding investment mistakes such as excessive risk.
The "Gamma" factor is an issue many investment experts have wrestled with for several years. Alpha and Beta are fairly straightforward to quantify but how do you begin to quantify "Gamma"?
Two investment experts from investment data provider Morningstar, David Blanchett, head of retirement research and Paul Kaplan, director of research, use a US study to try to quantify "Gamma" and look at the issues involved in assessing the true value of advice. Alpha and beta are usually seen at the heart of traditional performance analysis; however, as Mr Blanchett and Mr Kaplan show in this article, their impact on a successful retirement and successful investment can be far less important than other Financial Planning decisions made along the way.

Investment experts analyse the value of advice and its impact on long-term portfolio management success 
The potential benefits from "good" Financial Planning decisions are often difficult to quantify. For any given portfolio, investment decisions can generally be decomposed into two primary components: beta and alpha. Beta can generally be defined as the systematic risk exposures of the portfolio (usually achieved through asset allocation), while alpha is the residual, or skill/luck-based, component associated with the various flavours of active management (for example, tactical asset allocation, security selection and so on). Alpha and beta are at the heart of traditional performance analysis; however, as we show in this article, their impact on a successful retirement can be far less important than other Financial Planning decisions.
In this article we introduce a new concept called "Gamma" designed to measure the additional expected retirement income achieved by an individual investor from making more intelligent Financial Planning decisions. Gamma is the third letter in the Greek alphabet (preceded by alpha and beta) and within financial economics is sometimes used as the variable denoting the investor's degree of risk aversion. Given that Gamma is relatively unclaimed within the financial literature; we seek to give it a new meaning. Gamma varies for different investors as well as for investors in different lifecycles (for example, accumulation versus retirement). For those that find it hard to break from traditional (and inadequate) performance measurement, Gamma is a metric that is somewhat comparable to an alpha, or excess return but it is the return an investor experiences based on optimal decision making.
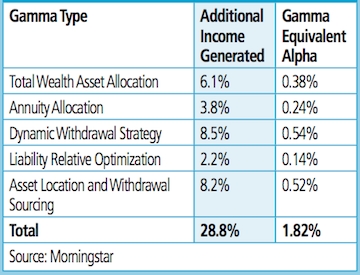
We focus on five important Financial Planning decisions/techniques: a total wealth framework to determine the optimal asset allocation, a dynamic withdrawal strategy, incorporating guaranteed income products (that is, annuities), tax-efficient allocation decisions, and a portfolio optimisation that includes the liability. Each of these five Gamma components creates value for retirees and, when combined, can be expected to generate 29 per cent more income on a utility-adjusted basis when compared to a simplistic static withdrawal strategy, according to our analysis. This additional income is equivalent to an arithmetic "alpha" of 1.82 per cent (that is, Gamma equivalent alpha) and represents a significant potential increase in portfolio efficiency (and retirement income) for retirees.

Gamma Factors
We've also examined in detail the potential long term value, or Gamma, that can be obtained from making "intelligent" Financial Planning decisions during retirement. A retiree faces a number of risks during retirement, some of which are unique to retirement planning and are not concerns during accumulation. We will explore five Gamma factors:
Total Wealth Asset Allocation: Using human capital in conjunction with the market portfolio to determine the optimal equity allocation. Most techniques used to determine the asset allocation for a client are relatively subjective and focus primarily on risk preference (that is, an investor's aversion to risk) and ignore risk capacity (that is, an investor's ability to assume risk). In practice, however, we believe asset allocation should be based on a combination of risk preference and risk capacity, although primarily risk capacity.
We determine an investor's risk capacity by evaluating his or her total wealth, which is a combination of human capital (an investor's future potential savings) and financial capital. We can then either use the market portfolio as the target aggregate asset allocation for each investor (as suggested by the Capital Asset Pricing Model) or build an investor-specific asset allocation that incorporates an investor's risk preferences. In both approaches, the financial assets are invested, subject to certain constraints, in order to achieve an optimal asset allocation that takes both human and financial capital into account.
Dynamic Withdrawal Strategy: The majority of retirement research has focused on static withdrawal strategies where the annual withdrawal during retirement is based on the initial account balance at retirement, increased annually for inflation. For example, a "four per cent Withdrawal Rate" would really mean a retiree can take a four per cent withdrawal of the initial portfolio value and continue withdrawing that amount each year, adjusted for inflation. If the initial portfolio value was $1 million and the withdrawal rate was four per cent, the retiree would be expected to generate $40,000 in the first year. If inflation during the first year was three per cent, the actual cashflow amount in year two (in nominal terms) would be $41,200. Under this approach the withdrawal amount is based entirely on the initial income target and is not updated based on market performance or expected investor longevity. The approach we use in this paper determines the annual withdrawal amount annually based on the ongoing likelihood of portfolio survivability and expected mortality.

{desktop}{/desktop}{mobile}{/mobile}
Annuity Allocation: Outliving one's savings is perhaps the greatest risk for retirees. A study by Allianz Life noted that the greatest fear among retirees is not death (39 per cent ) but rather outliving one's resources (61 per cent ). Annuities allow a retiree to hedge away this risk and can therefore improve the overall efficiency of a retiree's portfolio. The contribution of an annuity within a total portfolio framework, (benefit, risk, and cost) must be considered before determining the appropriate amount and annuity type.
Asset Location and Withdrawal Sourcing: Tax- efficient investing for a retiree can be thought of in terms of both "asset location" and intelligent withdrawal sequencing from accounts that differ in tax status. Asset location is typically defined as placing (or locating) assets in the most tax-efficient account type. For example, it generally makes sense to place less tax-efficient assets (that is, those where the majority of total return comes from coupons/ dividends taxed as ordinary income), such as bonds, in retirement accounts (for example, in the US, IRAs or 401ks) and more tax-efficient assets (that is, those where the majority of total return comes from capital gains taxed at a rate less than ordinary income), such as stocks, in taxable accounts. When thinking about withdrawal sequencing, it typically makes sense to withdrawal monies from taxable accounts first and more tax-efficient accounts (for example, IRAs or 401ks) later.
Liability-Relative Optimization: Asset allocation methodologies commonly ignore the funding risks, like inflation and currency, associated with an investor's goals. By incorporating the liability into the portfolio optimisation process it is possible to build portfolios that can better hedge the risks faced by a retiree. While these "liability-driven" portfolios may appear to be less efficient asset allocations when viewed from an asset-only perspective, we find hey are actually more efficient when it comes to achieving a sustainable retirement income.
From a more holistic perspective, each of these Gamma concepts can be thought of as actions and services provided by Financial Planners. In the interests of space, the actual Gamma calculation is not introduced in this piece (please contact the authors for the complete version). For the calculation we use an intertemporal substation parameter that is distinct from the risk tolerance parameter. We introduce the risk tolerance parameter next by treating the path as unknown and evaluating expected utility. The Gamma of strategy is defined as the increase the utility- adjusted income generated from a given strategy over a benchmark, which is assumed to be a 20 per cent equity portfolio with four per cent initial withdrawal rate for the purposes of this analysis.

Gamma Results
In this section we will review the relative impact of five different kinds of Gamma: using total wealth to determine the optimal asset allocation, a dynamic withdrawal strategy, incorporating guaranteed income products, tax-efficient allocation decisions, and liability-relative portfolio optimisation. If we add the results from the five different types of Gamma tested, we find a Gamma of 28.8 per cent, that is, $1.29 for every $1 generated by the base set of assumptions. Figure 1 shows the incremental and total income generated by each Gamma test.
An increase in certainty-equivalent utility- adjusted income of 28.8 per cent is an impressive improvement in potential retirement income, but how does it relate from a traditional alpha perspective? In order to determine how much additional annual return, or alpha, is equivalent to the 28.8 per cent Gamma, we conducted additional analysis. We determined the total income generated for a four per cent initial withdrawal rate and compare it to the income generated for portfolios with returns that are either higher or lower than the base portfolio by -2 per cent , -1 per cent , 0 per cent (no change), +1 per cent , +2 per cent , and +3 per cent. We compare the difference (Figure 2) in the amount of income generated against the income generated by the zero per cent change (that is, no change) portfolio. By fitting a third-order polynomial to the curve depicting the four per cent initial withdrawal, we estimate the equivalent annual return impact of a +28.8 per cent increase in retirement income to be 1.82 per cent . Table 1 shows how we attribute this Gamma- equivalent alpha among the five Gamma factors. This is likely to be significantly higher than any type of portfolio "alpha" that a Financial Planner would be able to generate through fund selection or market timing. Also, while traditional portfolio alpha is a negative-sum game, our results can be achieved by any investor who takes a smarter approach to generating retirement income.

Conclusion
In the aggregate, we estimate a retiree can be expected to generate 29 per cent more income on a certainty-equivalent, utility-adjusted basis utilising a Gamma-efficient retirement income strategy when compared to our base scenario, of a four per cent withdrawal rate and a 20 per cent equity allocation portfolio. This additional income is equivalent to an average annual return increase of +1.82 per cent (that is, Gamma-equivalent alpha), which represents a significant improvement for a retiree.
Unlike traditional alpha, which is likely a negative-sum game after fees, we find that Gamma (and Gamma-equivalent alpha) can be achieved by anyone following an efficient Financial Planning strategy.